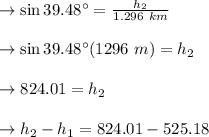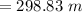Question

Two unidentified flying discs are detected by a receiver. The angle of elevation from the receiver to each disc is 39.48 degree. The discs are hovering at a direct distance of 826m and 1.296km from the receiver. Find the difference in height between the two unidentified flying discs, to the nearest meter.

Answer 1

The answer is "298.83 m"

Explanation:

Its gap from its transmitter from each disc comprises of 2 triangles. Its horizontal segment via disc to vertical, and longitudinal segment. Disk height, h, is parallel to a horizontal disc Wink including its line across recipient to disc or horizontal 39. 48^{\circ}

`\to 39. 48^(\circ) = (h_1)/(826 \ m)\\\\ \to 826\ m (\ sin 39. 48^(\circ) ) = h_1\\\\ \to 525.18 = h_1\\\\`

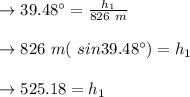
if:

`\to \sin 39. 48^(\circ) = (h_2)/(1.296 \ km)\\\\ \to \sin 39. 48^(\circ) (1296 \ m) = h_2\\\\ \to 824.01 = h_2\\\\\to h_2 - h_1 = 824.01 - 525.18\\\\`

`= 298.83 \ m`