Question

Point AAA is at {(6, -6)}(6,−6)left parenthesis, 6, comma, minus, 6, right parenthesis and point CCC is at {(-6,-2)}(−6,−2)left parenthesis, minus, 6, comma, minus, 2, right parenthesis. Find the coordinates of point BBB on \overline{AC} AC start overline, A, C, end overline such that AB=\dfrac34ACAB= 4 3 ​ ACA, B, equals, start fraction, 3, divided by, 4, end fraction, A, C.

Answer 1

Answer: (-3, -3)

Step-by-step explanation: Khan academy

Answer 2

B(-3, -3)

Explanation:

If a point O(x, y) divides line segment XY in the ratio of n:m and the endpoints of the segment are
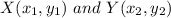`X(x_1,y_1)\ and\ Y(x_2,y_2)`, the coordinates of O is:

`x=(n)/(n+m)(x_2-x_1)+x_1 \\\\y=(n)/(n+m)(y_2-y_1)+y_1`

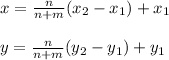
Given that A(6, -6) and C(-6, 2). Pont B is on AC such that:

AB = (3/4)AC

AB/AC = 3/4

Therefore point B divides the line AC in the ratio of 3:1. Let point B be at (x, y), therefore:

`x=(3)/(3+1)(-6-6)+6=(3)/(4)(-12)+6=-9+6=-3\\ \\y=x=(3)/(3+1)(-2-(-6))-6=(3)/(4)(4)-6=3-6=-3`

Therefore the location of B is at (-3, -3)