Question

Use the drawing tools to form the correct answer on the graph. Draw a point that belongs to the solution region of this system of inequalities.

Answer 1

#1

• y>1.5^x+4

Find y intercept

• y>1+4
• y>5

#2

• y<2/3x+6

Y intercept

• y<6

Both graphs attached

An point should be (1,6)

Answer 2

< or > = dashed line

≤ or ≥ = solid line

< or ≤ = shade below the line

> or ≥ = shade above the line

To draw:

`y < (2)/(3)x+6`

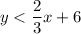
Treat as a linear equation.

Find 2 points:

`x=0 \implies y=(2)/(3)(0)+6=6 \implies (0,6)`

`x=3 \implies y=(2)/(3)(3)+6=8 \implies (3,8)`

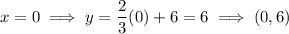
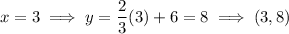
Plot the 2 points and draw a dashed straight line through them.

To draw:

`y > 1.5^x+4`

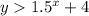
Treat as an exponential equation.

Find y-intercept:

`x=0\implies y=1.5^0+4=5\implies (0,5)`

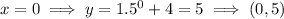
End behaviors:

`\textsf{As } x \rightarrow \infty, f(x) \rightarrow \infty`

`\textsf{As } x \rightarrow - \infty, f(x) \rightarrow 4`

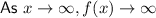
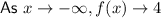
Draw the curve as a dashed line.

Once both lines are drawn, shade the overlapping region below the linear inequality and above the exponential inequality.

A point that belongs to the solution is any point in the shaded area,
e.g. (1, 6)