Question

A triangle has sides with lengths of 18 inches, 24 inches, and 30 inches. Is it a right triangle?

Answer 1

Yes

Explanation:

18^2+24^2=900

Square root of 900 is 30

This is derived from Pythagorean Theorem

Answer 2

It is a right triangle

Explanation:

Right Triangle

A right triangle has one of its internal angles of 90°. If such triangles, Pythagora's theorem must stand.

Being a and b the shortest sides of the triangle and c the longest side (hypotenuse), then:

`a^2+b^2=c^2`

The shortest sides of the given triangle are 18 and 24, and the longest side is 30, thus the following condition must be met to prove it's a right triangle:

`18^2+24^2=30^2`

`324+576=900`

`900=900`

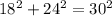
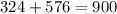
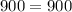
Since the condition is met, we can conclude it is a right triangle