Question

Can someone do this for me please?! Just the answer pls

Answer 1

See below for answers and explanations

Explanation:

Problem A

The equation of a circle is
`(x-h)^2+(y-k)^2=r^2` where
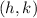`(h,k)` is the center and
`r` is the radius, thus, we need to find
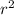`r^2` using our center and given point, through which we can find our equation:

`(x-h)^2+(y-k)^2=r^2\\\\(0-3)^2+(-2-6)^2=r^2\\\\(3)^2+(-8)^2=r^2\\\\9+64=r^2\\\\73=r^2`

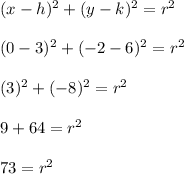
This means that the correct equation is
`(x-3)^2+(y-6)^2=73`

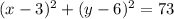
Problem B

`(x-h)^2+(y-k)^2=r^2\\\\(x-(-4))^2+(y-(-2))^2=9^2\\\\(x+4)^2+(y+2)^2=81`

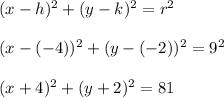
Thus, the correct equation is
`(x+4)^2+(y+2)^2=81`

Problem C

Use the distance formula where
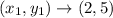`(x_1,y_1)\rightarrow(2,5)` and
`(x_2,y_2)\rightarrow(-10,7)` to find the diameter of the circle with the given endpoints:

`d=√((y_2-y_1)^2+(x_2-x_1)^2)\\\\d=√((7-5)^2+(-10-2)^2)\\\\d=√((2)^2+(-12)^2)\\\\d=√(4+144)\\\\d=√(148)\\\\d=2√(37)`

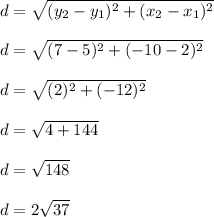
Since the radius is half the diameter, then
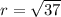`r=√(37)`, making
`r^2=37`.

The midpoint of the two endpoints will give us the center, so the center is
`\displaystyle \biggr((x_1+x_2)/(2),(y_1+y_2)/(2)\biggr)=\biggr((2+(-10))/(2),(5+7)/(2)\biggr)=\biggr((-8)/(2),(12)/(2)\biggr)=(-4,6)`

Thus, the correct equation is
`(x-h)^2+(y-k)^2=r^2\rightarrow(x-(-4))^2+(y-6)^2=37\rightarrow(x+4)^2+(y-6)^2=37`

Answer 2

a. center (3,6) –> (h,k)

point (0,-2) –> (x,y)

(x-h)²+(y-k)²=r²

(0-3)²+(-2-6)²=r²

(-3)²+(-8)²=r²

9+64=r²

r²=73

(x-h)²+(y-k)²=r²

(x-3)²+(y-6)²=73

____o_o___

b. center (-4,-2) –> (h,k)

radius =9 –> r

(x-h)²+(y-k)²= r²

(x-(-4))²+ (y-(-2))²= 9²

(x+4)²+(y+2)²=81

____o_o___

c. (2,5) –> (x1 , y1)

(-10,7) –> (x2,y2)

`( (x1 + x2 )/(2) , (y1 + y2)/(2) ) \\ \\ ( (2 + ( - 10))/(2) ,(5 + 7)/(2) ) \\ \\ ( ( - 8)/(2) , (12)/(2) ) = ( - 4,6)`

center (-4,6) –> (h,k)

(2,5) –> (x, y)

`{(x - h)}^(2) + (y - k)^(2) = {r}^(2) \\ (2 - ( - 4)) ^(2) + {(5 - 6)}^(2) = {r}^(2) \\ ({6})^(2) + {( - 1)}^(2) = {r}^(2) \\ 36 + 1 = {r}^(2) \\ {r}^(2) = 37`

`{(x - h)}^(2) + {(y - k)}^(2) = {r}^(2) \\ (x - ( - 4)) ^(2) + {(y - 6)}^(2) = {r}^(2) \\ (x + 4)^(2) + {(y - 6)}^(2) = 37`