Answer:
P = 43.3 units
Explanation:
the area (A) of the triangle is calculated as
A =
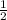 bh ( b is the base and h the height )
bh ( b is the base and h the height )
given A = 56 units²
with b =
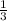 h
h
then
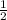 bh = 56 ( multiply both sides by 2 to clear the fraction )
bh = 56 ( multiply both sides by 2 to clear the fraction )
bh = 112 , so
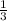 h × h = 112 ( multiply both sides by 3 to clear the fraction )
h × h = 112 ( multiply both sides by 3 to clear the fraction )
h² = 336 ( take square root of both sides )
h =
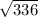 ≈ 18.3
≈ 18.3
then b =
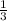 h =
h =
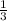 × 18.3 = 6.1
× 18.3 = 6.1
the height h from the vertex to the base bisects the base ( altitude ), that is
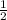 b =
b =
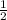 × 6.1 = 3.05
× 6.1 = 3.05
this divide the isosceles triangle into 2 right triangles
let the leg of the triangle be x
then using Pythagoras' identity in the right triangle formed
x² = 18.3² + 3.05² = 334.89 + 9.3 = 334.19 ( take square root of both sides )
x =
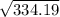 ≈ 18.6
≈ 18.6
Then perimeter (P) is
P = 2x + b = 2(18.6) + 6.1 = 37.2 + 6.1 = 43.3 units