Question

HURRY PLS 30 MINS LEFT

A ball is rolling across the floor so that it is traveling along the positive x axis at 5.00 m/s. Due to the bend of the carpet fibers it experiences an acceleration of 1.25 m/s2 at an angle of -125.0o from the x axis.


A. What is the x component of the acceleration?


B. What is the y component of the acceleration?


C. What is the x component of the balls displacement after 3.00s?


D. What is the y component of the balls displacement after 3.00s?


E. What is the displacement of the ball over 3.00s?

Answer 1

A) aₓ = -0.717 m / s² , B) a_{y} = -1.024 m / s², C) x = 11.77 m , D) y = -4.6 m ,

E) d = 12.64 m

Step-by-step explanation:

For this exercise we will use trigonometry to decompose the acceleration, we are told that

a = 1.25 m /s² with an angle tea = 125º in a clockwise direction

A) cos θ = aₓ / a

aₓ = a cos θ

aₓ = 1.25 cos (-125)

aₓ = -0.717 m / s²

B) sin θ =
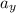`a_(y)` / a

a_{y} = a sin θ

a_{y} = 1.25 sin (-125)

a_{y} = -1.024 m / s²

C) The displacement component

x = v₀ₓ t + ½ aₓ t²

let's calculate

x = 5 3 + ½ (-0.717) 3²

x = 11.77 m

D) the y component of the displacement

y =
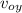`v_(oy)` t + ½
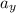`a_(y)` t²

in this case the initial velocity is zero

y = 0 + ½ (-1,024) 3²

y = -4.6 m

E) Let's use the Pythagorean theorem

d =√ (x² + y²)

d = √ (11.77² + 4.6²)

d = 12.64 m