Question

Given collinear points E, F and G such that point F is the midpoint of segment EG. Find the new length of EG given that EF =5x+9 and FG = 3x +17

Answer 1

The length of EG is 58 units

Explanation:

The midpoint of a segment divides it into two equal part

Let us use this rule to solve our question

∵ E, F, and G are collinear points

∵ Point F is the midpoint of segment EG

→ That means F divides EG into 2 equal segments EF and FG

∴ EF = FG

∵ EF = 5x + 9

∵ FG = 3x + 17

→ Equate them

∴ 5x + 9 = 3x + 17

→ Subtract 3x from both sides

∵ 5x - 3x + 9 = 3x - 3x + 17

∴ 2x + 9 = 17

→ Subtract 9 from both sides

∴ 2x + 9 - 9 = 17 - 9

∴ 2x = 8

→ Divide both sides by 2 to find x

∵
`(2x)/(2)=(8)/(2)`

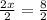
∴ x = 4

→ Substitute x by 4 in EF and FG to find their lengths

∵ EF = 5(4) + 9 = 20 + 9 = 29

∵ FG = 3(4) + 17 = 12 = 17 = 29

∵ EG = EF + FG

∴ EG = 29 + 29 = 58

∴ The length of EG is 58 units