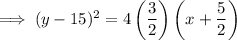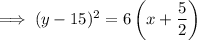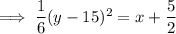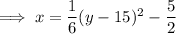Question

Given the focus (-1, 15) and the directrix x = -4, what is the equation of the parabola?

Answer 1

Answer: DE NADA

Step-by-step explanation: ANSWER EDMENTUM/PLUTO

Answer 2

`\textsf{C.} \quad x=(1)/(6)(y-15)^2-(5)/(2)`

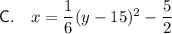
Explanation:

As the directrix is vertical, the parabola is sideways.

Conic form of a sideways parabola with a horizontal axis of symmetry:

`(y-k)^2=4p(x-h)\quad \textsf{where}\:p\\eq 0`

• Vertex = (h, k)
• Focus = (h + p, k)
• Directrix: x = (h - p)
• Axis of symmetry: y = k

If p > 0, the parabola opens to the right, and if p < 0, the parabola opens to the left.

Given:

• Focus: (-1, 15)
• Directrix: x = -4

Therefore:

• k = 15
• h + p = -1
• h - p = -4

Add h + p = -1 to h - p = -4 to eliminate p:

⇒ 2h = -5

⇒ h = -5/2

⇒ p = 3/2

Substituting the found values into the formula:

`\implies (y-15)^2=4\left((3)/(2)\right)\left(x+(5)/(2)\right)`

`\implies (y-15)^2=6\left(x+(5)/(2)\right)`

`\implies (1)/(6)(y-15)^2=x+(5)/(2)`

`\implies x=(1)/(6)(y-15)^2-(5)/(2)`