Question

1 What is the nature of the roots of the quadratic equation if the value of its discrimman

is zero?
The roots are not real
o the roots are rational and not equal
b. The roots are irrational and not equal d the roots are rational and equal
2. Whal is the ans of symmetry of the parabola y​

Answer 1

If d is zero, both roots are rational and equal.

Explanation:

Quadratic Equations

The standard representation of a quadratic function is:

`f(x)=ax^2+bx+c`

where a,b, and c are constants.

Solving with the quadratic formula:

`\displaystyle x=(-b\pm √(b^2-4ac))/(2a)`

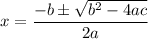
The discriminant is defined as:

`d=b^2-4ac`

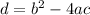
The sign of the discriminant defines the nature of the roots of the quadratic equation as follows:

• If d is positive, both roots are real and different
• If d is negative, both roots are imaginary and conjugate
• If d is zero, both roots are rational and equal.