Consider the traingle as per the attachment
In
 ADB. By Pythagoras theorem:
ADB. By Pythagoras theorem:
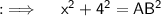
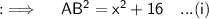
Now, In
 ADC. By Pythagoras theorem:
ADC. By Pythagoras theorem:

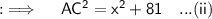
Now, In
 BAC. By Pythagoras theorem:
BAC. By Pythagoras theorem:
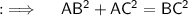
By (i) and (ii)
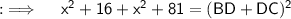
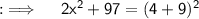
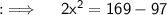
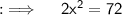
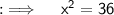
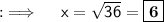
Hence, Option b) is correct
Pythagoras theorem:- It states that, in a right angled triangle the square of hypotenuse is equal to the sum of the square of base and square of perpendicular