Answer:
The average electrical power dissipated in the process is 0.653 mW
Step-by-step explanation:
Given;
gauge of copper wire, 20 gauge
resistivity from chart,

radius of the circular loop, r = 25 cm
magnetic field strength, B = 10 .0 mT
time, t = 0.34 s
Length of the wire, L = 2πr = 2 x π x 0.25 = 1.571 m
Area of the wire, A = πR² ⇒ R = D/2 = 0.8118 mm/ 2 = 0.4059 mm
= π(0.4059 x 10⁻³)² = 0.5177 x 10⁻⁶ m²
The resistance of the wire is given by;
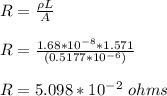
Now, determine the electric potential;

The average power is given by;
P = V²/R
P = (0.00577²) / (5.098 x 10⁻²)
P = 6.53 x 10⁻⁴ W
P = 0.653 mW
Therefore, the average electrical power dissipated in the process is 0.653 mW