Question

Suppose a star the size of our Sun, but with mass 9.0 times as great, were rotating at a speed of 1.0 revolution every 7.0 days. If it were to undergo gravitational collapse to a neutron star of radius 13 km , losing three-quarters of its mass in the process, what would its rotation speed be

Answer 1

Its rotation will be 3.89x10⁴ rad/s.

Explanation:

We can find the rotation speed by conservation of the angular momentum:

`L_(i) = L_(f)`

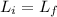
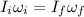`I_(i)\omega_(i) = I_(f)\omega_(f)` (1)

The initial angular speed is:

`\omega_(i) = (1 rev)/(7 d) = 0.14 (rev)/(d)`

The moment of inertia (I) of a sphere is:

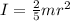`I = (2)/(5)mr^(2)` (2)

Where m is 9 times the sun's mass and r is the sun's radius

By entering equation (2) into (1) we have:

`(2)/(5)m_(i)r_(i)^(2)\omega_(i) = (2)/(5)m_(f)r_(f)^(2)\omega_(f)`

`9m_(sun)(696342 km)^(2)0.14(rev)/(d) = (3)/(4)9m_(sun)(13 km)^(2)\omega_(f)`

`\omega_(f) = (4)/(3)*0.14 (rev)/(d)((696342 km)/(13 km))^(2) = 5.36 \cdot 10^(8) (rev)/(d)*(1 d)/(24 h)*(1 h)/(3600 s)*(2\pi rad)/(1 rev) = 3.89 \cdot 10^(4) rad/s`

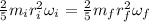
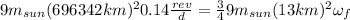
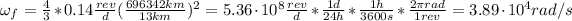
Hence, its rotation will be 3.89x10⁴ rad/s.

I hope it helps you!