Answer:
The final displacement from the origin is 1.6 km to the NE
Step-by-step explanation:
The directions in which the delivery truck travels are;
1) 2.8 km North = 2.8·
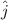 , in vector form
, in vector form
2) 1.0 km East = 1.0·
 , in vector form
, in vector form
3) 1.6 km South = -1.6·
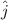 , in vector form
, in vector form
Therefore, to find the final displacement, Δx, of the delivery truck, we add the individual displacements as follows;
Final displacement, Δd = 2.8·
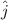 + 1.0·
+ 1.0·
 +(-1.6·
+(-1.6·
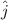 ) = 1.2·
) = 1.2·
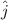 + 1.0·
+ 1.0·

Final displacement, = 1.0·
 + 1.2·
+ 1.2·
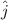
Where;
Δx = The displacement in the x-direction = 1.0·

Δy = The displacement in the y-direction = 1.2·
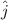
The magnitude of the resultant displacement vector is given as follows
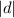 = √((Δx)² + (Δy)²) = √(1² + 1.2²) ≈ 1.6 (To the nearest tenth)
= √((Δx)² + (Δy)²) = √(1² + 1.2²) ≈ 1.6 (To the nearest tenth)
The magnitude of the resultant displacement vector ≈ 1.6 km
The direction of the resultant vector is positive for both the east and north direction, therefore, the direction of the resultant vector = NE
Therefore, the resultant displacement of the delivery truck is approximately 1.6 km, NE from the origin.