9514 1404 393
Answer:
a) take it back to the author for clarification
b) use a graphing calculator
c) mark known points and draw the curve
Explanation:
In the normal course of interpreting an algebraic expression, the evaluation of functions generally takes precedence over other operations. Here, that means this should be interpreted as ...
3·sin(1/2)·(x-1) +5
This is a (somewhat unusual) linear equation. It can be graphed by evaluating it for a couple of different x-values and drawing a line through the result. The first attachment shows a table of values and a graph of the line. (The slope of the line is an irrational value near 1.4382766 ≈ 105/73.)
__
It is common in Algebra studies to look at scaled and shifted versions of different functions. An alternative interpretation of the given expression would locate parentheses so that the sine function argument is clear:
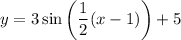
This is the equation of a sine function vertically expanded by a factor of 3, vertically shifted by 5 units, horizontally expanded by a factor of 2, and horizontally shifted by 1 unit.
This can be graphed a couple of ways.
Relabel Axes
A way to do it is to draw a sine function (amplitude 1, period 2π, no vertical or horizontal shift), then relabel the axes so the function center-line is at y=5, the peak values are at +3 and -3 from that line, the period is 4π, and the whole graph is shifted right so the first upward center-line crossing is at x=1.
Choose Points
Perhaps an expected way to graph the function is to choose several values for x, find the corresponding y-values, and draw the curve through the points.
Recompute Points
Rather than relabel the axes or choosing random points, you could start with an (x, y) table of sine function values, then "adjust" those values as follows:
each x-value: multiply by 2 and add 1
each y-value: multiply by 3 and add 5
Plot the "adjusted" value pairs, and draw a curve through them. See the second attachment for a graph.
__
Comment on graphing
My preference for graphing anything that involves an electronically transmitted result or any detailed calculation is to use "technology". My current preference is a graphing calculator. Spreadsheet programs can also do a decent job of graphing, given enough points to work with.