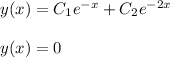Question

Solve the differential equation y" + 3y' + 2y = P1 where, y" = d2y/dx2, y' = dy/dx, P1 = P1(x)) and the initial conditions are y(0) = 0, y'(0) = 0. The input function is given by P1() = { 0, < 0 1, 0 ≤ ≤ 1 0, > 1

Answer 1

`y(x) = 0`

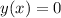
Explanation:

Let P(1) = 0, the differential equation becomes:

y" + 3y' + 2y = 0

Also let y' = my and y'' = m²y

Substitute

m²y+3my+2y = 0

(m²+3m+2)y = 0

The auxiliary equation is expressed as:

m²+3m+2 = 0

Factorize

m²+2m+m+2 = 0

m(m+2)+1(m+2) = 0

(m+1)(m+2) = 0

m+1 = 0 and m+2 = 0

m₁ = -1 and m₂ = -2

Since the value of m is real and distinct, the solution to the differential equation will be expressed as:

`y = C_1e^(m_1x)+C_2e^(m_2x)\\`

`y(x) = C_1e^(-x)+C_2e^(-2x)\\`

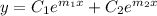
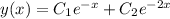
Given the initial condition y(0) = 0

`y(0) = C_1e^(-0)+C_2e^(-2(0))\\0 = C_1+C_2\\C_1+C_2 = 0 ................. 1`

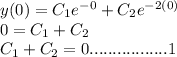
If y'(0) = 0

`y'(x) = -C_1e^(-x)-2C_2e^(-2x)\\y'(0) = -C_1e^(-0)-2C_2e^(-2(0))\\0 = -C_1-2C_2\\C_1-2C_2 = 0...................... 2`

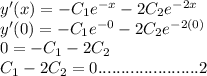
Solve eqn 1 and 2 simultaneously

From 1:

C₁ = -C₂ .......... 3

Substitute equation 3 into 2:

-C₂-2C₂ = 0

-3C₂ = 0

C₂ = 0

Substitute the constant into the differential equation.

`y(x) = C_1e^(-x)+C_2e^(-2x)\\\\y(x) = 0`