Question

A 6.16-g bullet is moving horizontally with a velocity of 342 m/s, where the sign indicates that it is moving to the right (see part a of the drawing). The bullet is approaching two blocks resting on a horizontal frictionless surface. Air resistance is negligible. The bullet passes completely through the first block (an inelastic collision) and embeds itself in the second one, as indicated in part b. Note that both blocks are moving after the collision with the bullet. The mass of the first block is 1155 g, and its velocity is 0.728 m/s after the bullet passes through it. The mass of the second block is 1517 g.

(a) What is the velocity of the second block after the bullet imbeds itself?
(b) Find the ratio of the total kinetic energy after the collisions to that before the collisions.

Answer 1

a

The value is
`v = 0.8351 \  m/s`

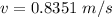
b

The ratio is
`(K_a)/(K_b)  =   5.94 11 *10^(-6)`

Step-by-step explanation:

From the question we are told that

The mass of the bullet is
`m_b  =  6.16 \  g =  0.00616 \  kg`

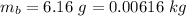
The velocity is
`u_b  =  342 \  m/s`

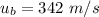
mass of the first block is
`m__{{B_1}}} =  1155 \  g  =  1.155 \  kg`

The velocity of the first block after bullet passes is
`v__{{B_1}}} =  0.728 m/s`

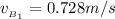
The mass of the second block is
`m__{{ B_2}}} = 1517 g =  1.517 \ kg`

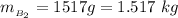
Gnerally according to the law of momentum conservation

`m_b *  u_b  +  m__{{B_1}}} *  u__{{B_1}}} = m__{{B_1}}}  *  v__{{B_1}}}  + v_b *  m_b`

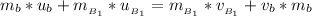
Here
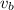`v_b` is the velocity of the bullet emerging from the first block

and
`u__{{B_1}}}` is zero because initial the first block was at rest

So

`0.00616 *  342  +  1.155*  0 =  v_b *  0.00616 +   1.155 *  0.728`

`v_b =  206.5 \  m/s`

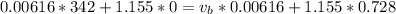
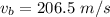
Considering the second block

Gnerally according to the law of momentum conservation

`m_b *  v_b +  m__{{B_2}}} *  u__{{B_2}}} = [m__{{B_2}}} +m_b] v`

Here
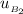`u__{{B_2}}}` is zero because initial the second block was at rest

=>
`0.00616 *  206.5  +  1.517*  0= [1.517 +m_b] v`

=>
`0.00616 *  206.5  = [1.517 +0.00616] v`

=>
`v = 0.8351 \  m/s`

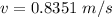
The kinetic energy of the bullet before collision is

`K_b  =  (1)/(2) *  m  *  u_b^2`

=>
`K_b  =  0.5  *  0.00616  *  342^2`

=>
`K_b  =  360.2 \  J`

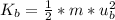
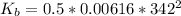
The kinetic energy of the bullet after collision is

`K_a  =  (1)/(2) *  m  *  v^2`

=>
`K_a  =  0.5  *  0.00616  *  0.8351^2`

=>
`K_a  =  0.00214 \  J`

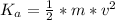
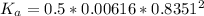
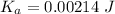
Generally the ratio of the kinetic energy is mathematically represented as

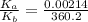
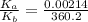
`(K_a)/(K_b)  =  (0.00214)/(360.2 )`

=>
`(K_a)/(K_b)  =  (0.00214)/(360.2 )`

=>
`(K_a)/(K_b)  =   5.94 11 *10^(-6)`