Question

A train starting from a distance of velocity of 90 km.h

-1 in 10 minutes assuming that the acceleration is uniform find the acceleration and the distance travelled by the train for attending the velocity​

Answer 1

To make this problem solvable and you can get the help you need, I'll complete and arrange some data.

Answer:

Acceleration:
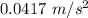`0.0417\ m/s^2`, Distance=7,500 m

Step-by-step explanation:

Uniform Acceleration Motion

It's a type of motion in which the velocity of an object changes uniformly over time.

Being a the constant acceleration, vo the initial speed, vf the final speed, and t the time, the following relation applies:

`v_f=v_o+at\qquad\qquad [1]`

The distance traveled by the object is given by:

`\displaystyle x=v_o.t+(a.t^2)/(2)\qquad\qquad [2]`

Using the equation [1] we can solve for a:

`\displaystyle a=(v_f-v_o)/(t)\qquad\qquad [3]`

The problem will be rewritten as follows:

A train starting from rest reaches a velocity of 90 km/h in 10 minutes. Assuming that the acceleration is uniform, find the acceleration and the distance traveled by the train for attending the velocity​.

Let's take the relevant data:

vo=0

vf=90 Km/h*1000/3600 = 25 m/s

t = 10 minutes = 10*60 = 600 seconds

Now compute the acceleration by using [3]:

`\displaystyle a=(25-0)/(600)=0.0417`

`a=0.0417\ m/s^2`

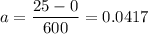
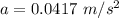
Finally, compute the distance:

`\displaystyle x=0*600+(0.0417\cdot 600^2)/(2)`

`x=7,500\ m`

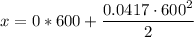
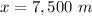
Note: We used the value of the acceleration with more precision than shown.

Acceleration:
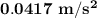`\mathbf{0.0417\ m/s^2}`, Distance=7,500 m