Question

In a right triangle, one leg (a) is 10 cm, and its hypotenuse (c) is 26 cm. Find b?​

Answer 1

`\boxed {\tt b=24 \ centimeters}`

Explanation:

This is a right triangle, so we can use Pythagorean Theorem.

`a^2+b^2=c^2`

a and b are the legs, while c is the hypotenuse.

One leg is 10 centimeters. The hypotenuse is 26 centimeters. Therefore,

`a= 10 \ cm \\c= 26 \ cm`

`(10 \ cm )^2+b^2=(26 \ cm)^2`

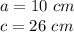
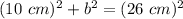
Solve the exponents.

• (10 cm)²= 10 cm * 10 cm= 100 cm²

`100 \ cm^2+b^2=(26 \ cm)^2`

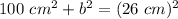
• (26 cm)²= 26 cm * 26 cm= 676 cm²

`100 \ cm^2+b^2=676 \ cm^2`

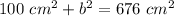
Subtract 100 from both sides of the equation.

`100 \ cm^2- 100 \ cm^2+b^2=676 \ cm^2- 100 \ cm^2`

`b^2=676 \ cm^2 - 100 \ cm^2`

`b^2= 576 \ cm^2`

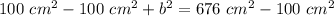
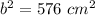
Take the square root of both sides of the equation.

`√(b^2) =√(576 \ cm^2)`

`b=√(576 \ cm^2)`

`b= 24 \ cm`

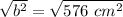
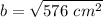
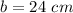
The other leg of the triangle is equal to 24 centimeters.