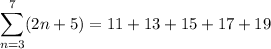Question

Sum n=3 ^ infty (2n+5)
find the series shown​

Answer 1

Answer: Choice C) 11 + 13 + 15 + 17 + ...

=====================================================

Step-by-step explanation:

We're adding terms in the form (2n+5)

The first term is when n = 3, so

2n+5 = 2*3+5 = 6+5 = 11

The next term is when n = 4, so

2n+5 = 2*4+5 = 8+5 = 13

The next term is when n = 5, so

2n+5 = 2*5+5 = 10+5 = 15

And so on

This means,

`\displaystyle \sum_(n=3)^(\infty) (2n+5) = 11+13+15+17+\ldots`

which matches with choice C. The three dots indicates the pattern goes on forever.

-------------------

Choice B does not have these dots, so that's why choice B is not the answer. If we replaced the infinity symbol with 7, then choice B would be the answer.

I.e,

`\displaystyle \sum_(n=3)^(7) (2n+5) = 11+13+15+17+19`