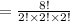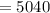Answer:
5 040 permutations
Explanation:
the word ‘HONOLULU’ has 8 letters
In this word ,the letters O , L and U are repeated each two times.
the number of permutations of the two letters O , o is 2!
the number of permutations of the two letters L , L is 2!
the number of permutations of the two letters U , U is 2!
the number of permutations (distinguishable or not) of the letters in HONOLULU is 8!
then
the number of distinguishable permutations of the letters in HONOLULU :