Answer:
The angle of depression from Platform A to Platform B is

Explanation:
Refer the attached figure
The horizontal distance between the platforms is 500 feet i.e. BC = 500
The length of the zip-line is 685 feet i.e. AB = 685
We are supposed to find the angle of depression from Platform A to Platform B
Hypotenuse = 685
Base = 500
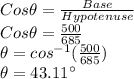
Hence the angle of depression from Platform A to Platform B is
