Question

Anne works for a company that manufactures liquid dyes for clothing. She currently wants to mix up some 30%-concentrated red dye. She has 66 gallons of 21%-concentrated red dye, as well as plenty of 51%-concentrated red dye. How many gallons of the 51%-concentrated red dye will Anne need to add to the 21%-concentrated red dye to make a batch with a concentration of 30%?

Answer 1

28.29 gallons

Explanation:

Given that the available amount of 21%-concentrated red dye= 66 gallons.

Let x gallons of 51%-concentrated red dyes to be mixed with 56 gallons of 21%-concentrated red dye to get 30%-concentrated red dyes.

Total amount of the resulting mixture = 66 + x gallons.

Amount of red dyes in the mixture = (21% of 66) + (51% of x)

To get the resulting mixture as 30%-concentrated red dyes, we have

30% of (66+x) = (21% of 66) + (51% of x)

`\Rightarrow 30(66+x)=21*66+51x\\ \Rightarrow 30* 66 +30x= 21*66+51x\\\Rightarrow 51x-30x=66(30-21)\\\Rightarrow 21x=66* 9\\\Rightarrow x = 28.29`

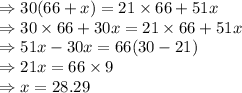
Hence, the amount of 51%-concentration red dyes to be mixed = 28.29 gallons.