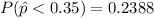Answer:
The probability is
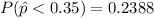
Explanation:
From the question we are told that
The population proportion is p = 0.40
The sample size is n = 50
Generally the standard error is mathematically represented as
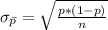
=>
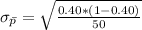
=>
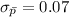
Generally the probability that a random sample of 50 U.S. Adults has less than 35% with this opinion is mathematically represented as
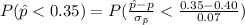
Generally

=>
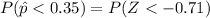
From the z table
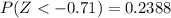
So