Question

A regional office of the Internal Revenue Service randomly distributes returns to be audited to the pool of auditors. Over the thousands of returns audited last year, the average amount of extra taxes collected was $356 per audited return. One of the auditors, Jeffrey Jones, is suspected of being too lenient with persons whose returns are being audited. For a simple random sample of 30 of the returns audited by Mr. Jones last year, an average of $340 in extra taxes was collected.

Required:
Assuming a normal population of extra taxes collected with a standard deviation of $90, do the suspicions against Mr. Jones appear to be justified?

Answer 1

The decision rule is

Fail to reject the null hypothesis

The conclusion is

There is sufficient evidence to conclude that the justification against Mr. Jones appears not to be justified

Explanation:

From the question we are told that

The population mean is
`\mu  =  \$ 356`

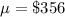
The sample size is n = 30

The sample mean is
`\= x = \$340`

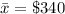
The standard deviation is
`\sigma =  \$ 90`

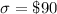
The null hypothesis is
`H_o  :  \mu =  \$ 35 6`

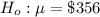
The alternative hypothesis is
`H_a  :  \mu \\e  \$ 35 6`

Let assume the significance level is
`\alpha = 0.05`

Generally the test statistic is mathematically represented as

`t = (\= x - \mu )/((\sigma )/(√(n) ) )`

=>
`t = ( 340 - 356 )/((90 )/(√(30) ) )`

=>
`t =-0.9737`

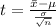
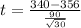
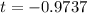
Generally the sample size from the question is small that it is not greater than 30 hence we use t-test

Now the degree of freedom is mathematically represented as

`df =  n- 1`

=>
`df =  30- 1`

=>
`df= 29`

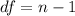
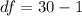
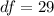
Generally the p-value is mathematically represented as

`p-value =  2 P (t >  -0.9737 )`

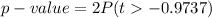
Generally from the t-distribution table , the probability of -0.9737 at a degree of freedom of
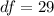`df= 29` for a two -tailed test is

`P (t >  -0.9737 ) =  0.33825793`

Hence p-value is

`p-value =  2*0.33825793`

`p-value =  0.676`

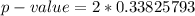
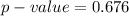
From the value we obtained we see that
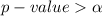`p-value  >  \alpha` hence

The decision rule is

Fail to reject the null hypothesis

The conclusion is

There is sufficient evidence to conclude that the justification against Mr. Jones appears not to be justified