Question

The results of a recent survey indicate that the average new car costs $23,000 with a standard deviation on $3,500. The prices of the car are normally distributed. a. If someone buys a car for $32,000, what proportion of cars cost an equal amount or more than this? b. If someone buys a car for $16,000, what proportion of cars cost an equal amount or more than this?

Answer 1

(a) 0.0051

(b) 0.9773

Explanation:

Let X = prices of the car.

It is provided that
`X\sim N(23,000, 3,500^(2))`.

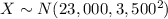(a)

Compute the value of P (X ≥ 32000) as follows:

`P(X\geq 32000)=P((X-\mu)/(\sigma)>(32000-23000)/(3500))`

`=P(Z>2.57)\\=1-P(Z<2.57)\\=1-0.99492\\=0.00508\\\approx 0.0051`

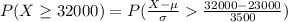
Thus, the proportion of cars cost an equal amount or more than $32,000 is 0.0051.

(b)

Compute the value of P (X ≥ 16000) as follows:

`P(X\geq 16000)=P((X-\mu)/(\sigma)>(16000-23000)/(3500))`

`=P(Z>-2)\\=P(Z<2)\\=0.97725\\\approx 0.9773`

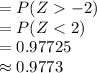
Thus, the proportion of cars cost an equal amount or more than $16,000 is 0.9773.