Question

One strategy in a snowball fight is to throw

a snowball at a high angle over level ground.
While your opponent is watching this first
snowball, you throw a second snowball at a
low angle and time it to arrive at the same
time as the first.
Assume both snowballs are thrown with
the same initial speed 16.2 m/s. The first
snowball is thrown at an angle of 62° above
the horizontal. At what angle should you
throw the second snowball to make it hit the
same point as the first? Note the starting and
ending heights are the same. The acceleration
of gravity is 9.8 m/s.

Answer 1

second question: How many seconds after the first snowball

should you throw the second so that they

arrive on target at the same time?

Answer in units of s.

Answer:

Part 1: 28°

Part 2: 1.367

Step-by-step explanation:

Part 1:

Given: 62°

Simple

θ = 90°- 62°

θ = 28°

Part 2:

Y-direction

Δy
`=v_(yo) t+(1)/(2) a_(y) t^(2)`

`0=[16.2sin(62)]t_(1)+1/2(-9.8)t_(1)^(2) \\`

`t_(1) =(2[16.2sin(62)])/(9.8)`

`t_(1)=2.91913s`

`0=[16.2sin(28)]t_(2)+1/2(-9.8)t_(2)^(2)`

`t_(2) =(2[16.2sin(28)])/(9.8)`

`t_(2)=1.55213s`

Δt
`=t_(1)-t_(2)`

Δt
`=2.91913-1.55213`

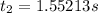
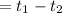
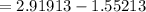
Δt= 1.367s

Hope it helps :)