Question

I really need help please just answer at least one

Answer 1

9) a = 25 [m/s^2], t = 4 [s]

10) a = 0.0875 [m/s^2], t = 34.3 [s]

11) t = 32 [s]

Step-by-step explanation:

To solve this problem we must use kinematics equations. In this way we have:

9)

a)

`v_(f)^(2) = v_(i)^(2)-(2*a*x)\\`

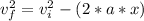
where:

Vf = final velocity = 0

Vi = initial velocity = 100 [m/s]

a = acceleration [m/s^2]

x = distance = 200 [m]

Note: the final speed is zero, as the car stops completely when it stops. The negative sign of the equation means that the car loses speed or slows down as it stops.

0 = (100)^2 - (2*a*200)

a = 25 [m/s^2]

b)

Now using the following equation:

`v_(f) =v_(i) - (a*t)`

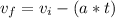
0 = 100 - (25*t)

t = 4 [s]

10)

a)

To solve this problem we must use kinematics equations. In this way we have:

`v_(f) ^(2) = v_(i) ^(2) + 2*a*(x-x_(o))`

Note: The positive sign of the equation means that the car increases his speed.

5^2 = 2^2 + 2*a*(125 - 5)

25 - 4 = 2*a* (120)

a = 0.0875 [m/s^2]

b)

Now using the following equation:

`v_(f)= v_(i)+a*t\\`

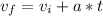
5 = 2 + 0.0875*t

3 = 0.0875*t

t = 34.3 [s]

11)

To solve this problem we must use kinematics equations. In this way we have:

`v_(f) ^(2) = v_(i) ^(2) + 2*a*(x-x_(o))`

10^2 = 2^2 + 2*a*(200 - 10)

100 - 4 = 2*a* (190)

a = 0.25 [m/s^2]

Now using the following equation:

`v_(f)= v_(i)+a*t\\`

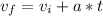
10 = 2 + 0.25*t

8 = 0.25*t

t = 32 [s]