Answer:
Multiply the first fraction by
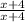
Multiply the second fraction by
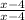
Explanation:
Given
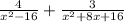
Required
Make the denominator equal
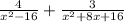
Factorize the denominator
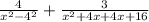

Multiply the first fraction by
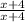
Multiply the second fraction by
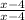
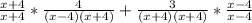
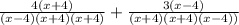
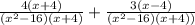
The expression now have the same denominator