Question

The annual profit at a chain of stores is normally distributed with a mean of $61,000 and a standard

deviation of $23,000. The stores in the top 5% of annual profit were rewarded with a celebration. What
was the annual profit required to have a party?

Answer 1

Answer: $98,835.

Explanation:

Given: Annual profit at a chain of stores is normally distributed with a mean (
`\mu`) of $61,000 and a standard deviation(
`\sigma`) of $23,000.

Let
`x` denoted the profit.

Let
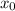`x_0` be the minimum profit required to have a party.

The stores in the top 5% (i.e. 0.05) of annual profit were rewarded with a celebration.

i.e.
`P(x>x_0)=0.05`

`\Rightarrow\ P((x-\mu)/(\sigma)>(x_0-61000)/(23000))=0.05\\\\\Rightarrow\ P(z>(x_0-61000)/(23000))=0.05\ \ \ [z=(x-\mu)/(\sigma)]\\\\\Rightarrow\ (x_0-61000)/(23000)=1.645\ \ [\text{critical z-value for p-value 0.05=1.645}]\\\\\Rightarrow\ x_0-61000 =1.645* 23000\\\\\Rightarrow\ x_0-61000 =37835\\\\\Rightarrow\ x_0=37835+61000\\\\\Rightarrow\ x_0=98835`

Hence, the annual profit required to have a party was $98,835.