Answer:
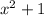 cannot be factored, thus it's a prime polynomial
cannot be factored, thus it's a prime polynomial
Explanation:
A prime number or expression cannot be factored in any factor different from 1 and the number or expression itself.
One of the following expressions is prime. The rest of them can be factored.
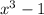 is the difference of two cubes. It can be factored as:
is the difference of two cubes. It can be factored as:
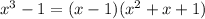
 is the sum of two cubes. It can be factored as:
is the sum of two cubes. It can be factored as:
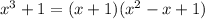
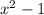 is the difference of two squares. It can be factored as:
is the difference of two squares. It can be factored as:

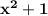 cannot be factored, thus it's a prime polynomial
cannot be factored, thus it's a prime polynomial