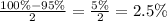Answer:
2.5%
Explanation:
According to the Empirical Rule:
- 68% of the data in a normal distribution are
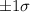 from the mean
from the mean
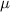
- 95% of the data in a normal distribution are
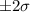 from the mean
from the mean
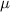
- 99.7% of the data in a normal distribution are
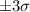 from the mean
from the mean
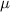
Hence, the percentage of boxes that have weights of more than 2 standard deviations below the mean is