Answer:
Between x = 48 and x = 45.
Explanation:
Average rate of change of a function in the given interval is represented by,
Average rate of change =
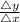
From the table attached,
Average rate of change of the function between x = 3 and x = 9
=
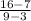
= 3
Average rate of change in the interval x = 9 and x = 22
=
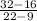
=

= 1.23
Average rate of change in the interval x = 9 and x = 22,
=
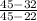
=

= 0.57
Average rate of change in the interval x = 45 and x = 48,
=
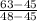
= 6
Therefore, average rate of change is maximum between x = 45 and 48.
Option given in bottom right will be the answer.