Question

Can someone please answer this

Answer 1

`\boxed{\begin{array}{ccc}1&5&4\\*&&3\\\cline{1-3}\vphantom{(1)/(2)}4&6&2\end{array}}`

Explanation:

You want to use the numbers 1–6 one time each to create the product of a 3-digit number with a 1-digit number such that the result is between 400 and 500.

Numbers

Let's designate the values using the letters ...

`\begin{array}{ccc}A&B&C\\*&&D\\\cline{1-3}\vphantom{(1)/(2)}4&E&F\end{array}`

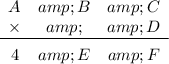
The value of D cannot be 1, because C ≠ F. It cannot be 2, because that would require A=D=2.

If D is 4, then A=1 and the product B·D must be less than 7, requiring B=1, which it cannot be.

This leaves D=3, A=1.

In order for the product D·C to have an appropriate value we must have C=2 or C=4. If C=2, then F=6 and we have used the digits 1, 2, 3, 6. There is no carry from 3·2 = 6, so B and E cannot be 4 and 5 (in either order).

That leaves C=4, F=2, and {B, E} = {5, 6}. The carry of 1 from the product D·C = 3·4 = 12 means that E = B+1, so B=5 and E=6.

Now we have ABC = 154, D=3, EF=62. The desired product is ...

`\boxed{\begin{array}{ccc}1&5&4\\*&&3\\\cline{1-3}\vphantom{(1)/(2)}4&6&2\end{array}}`

__

Additional comment

We have attached a multiplication table to help in visualizing the products. Any cell with a digit equal to 0 or greater than 6 cannot be used. Nor can any cell be used for C or D where a product digit matches a factor digit.

<95141404393>