Question

Plot a graph showing the variation of stopping potential with the frequency of incident radiation for two different photosensitive materials having work Functions
`{\omega{1}}` and
`{\omega{2}}`
`{(\omega{1}>\omega{2})}`. On what factors does the (i) Slope and (ii) intercept of the lines depends?​

Answer 1

We know By Einstein's Photoelectric Equation:

`{:\implies \quad \sf h\\u =h\\u_(0)+(1)/(2)m{v^(2)}_(max)}`

`{:\implies \quad \sf h\\u =h\\u_(0)+eV_(0)}`

`{:\implies \quad \sf eV_(0)=h\\u -h\\u_(0)}`

`{:\implies \quad \sf V_(0)=(h\\u)/(e)-(h\\u_(0))/(e)}`

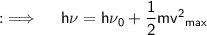
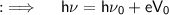
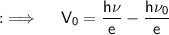
Since
`{\\u}` is plotted along X-axis and
`{V_0}` along Y-axis, it represents a straight line

(i) The slope of
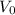`{V_(0)}` versus
`{\\u}` graph= (h/e) i.e it is a constant quantity and does not depend on the nature of the metal surface

(ii) The intercept on the
`{\\u}`-axis =
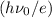`{(h\\u_(0)/e)}` i.e it depends on the work function of the metal surface

Refer to the attachment for the graph