Answer:
h = 61.25 m
Explanation:
It is given that,
The initial velocity of the ball, v = 60 m/s
It is thrown from a height of 5 feet,
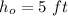
We need to find the maximum height it reaches. The height reached by the projectile as a function of time t is given by :

Putting all the values,
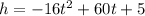 .....(1)
.....(1)
For maximum height, put
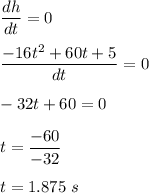
Put t = 1.875 in equation (1)
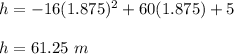
So, the maximum height reached by the ball is 61.25 m.