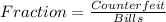Question

Show that each conjecture is false by finding a counterexample.

1.For any integer n, n3 > 0.


2. Each angle in a right triangle has a different measure.


3.For many years in the U.S, each bank printed its own currency. The variety of different bills led to widespread counterfeiting. By the time of the Civil War, a significant fraction of the currency in circulation was counterfeit. If one soldier had 48 bills, 16 of which were counterfeit, and another soldier had 39 bills, 13 of which were counterfeit, make a conjecture about what fraction of bills were counterfeit at the time of the Civil War.

Answer 1

1.
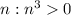`n: n^3 > 0` is not true for negative integers

2.
`45\ and\ 45` show that all angles do not have different measure

3.
`Fraction = (1)/(3)`

Explanation:

Solving (1):

`n: n^3 > 0`

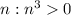
Take

`n = -1`

`-1^3 = -1`

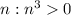`n: n^3 > 0` is not true for negative integers

Solving (2):

The angles of a right angled triangle are:

`45, 45\ and\ 90`

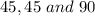
`45\ and\ 45` show that all angles do not have different measure

Solving (3):

When

`Bills = 48`

`Counterfeit = 16`

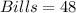
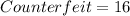
The fraction is calculated as thus:

`Fraction = (Counterfeit)/(Bills)`

`Fraction = (16)/(48)`

`Fraction = (1)/(3)`

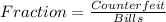
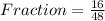
Similarly, when

`Bills = 39`

`Counterfeit = 13`

`Fraction = (Counterfeit)/(Bills)`

`Fraction = (13)/(39)`

`Fraction = (1)/(3)`