Question

An airplane pilot over the Pacific sights a ship wreck at an angle of depression of 5°. At this time, the horizontal distance from the airplane to the wreck is 4629 meters. What is the height of the plane to the nearest meter?

405 m

Answer 1

The height of the plane is 405 meters

Explanation:

Trigonometric Ratios

The situation can be represented as shown in the image below. The ground, the height H, and the direct distance to the plane to the shipwreck form a right triangle, where the trigonometric ratios stand.

Since the known distance is adjacent to the angle, and the required height is opposite to the given angle, we use the tangent ratio, defined as:

`\displaystyle \tan\ x=\frac{\text{opposite leg}}{\text{adjacent leg}}`

`\displaystyle \tan 5^\circ=(H)/(4629)`

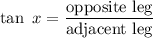
We need to find H, so we solve for H:

`H=4629\cdot\tan5^\circ`

H=405 m

The height of the plane is 405 meters