Question

Find the slope of the line that contains (3, –2) and (4, 3).
Can someone help fast please?

Answer 1

5

Explanation:

M equals slope

M=3- -2/4-3

M=5/1

Therefore your slope would be 5

Answer 2

`\boxed {\boxed {\sf m=5}}`

Explanation:

The slope of a line tells us the steepness and direction of a line. It is the change in y over the change in x (rise over run). It is calculated using the following formula:

`m= (y_2-y_1)/(x_2-x_1)`

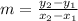
In this formula, (x₁, y₁) and (x₂,y₂) are the coordinates the line passes through. The line contains (3, -2) and (4,3), therefore:

• x₁ = 3
• y₁ = -2
• x₂ =4
• y₂ = 3

Substitute the values into the formula.

`m= (3 --2)/(4-3)`

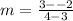
Solve the numerator.

• 3 --2 = 3+2 = 5

`m= (5)/(4-3)`

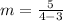
Solve the denominator.

`m= (5)/(1)=5`

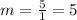
The slope of the line is 5.