Answer:
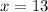
Explanation:
Step 1: Isolate the square root on the left hand side
Radical already isolated
√x-4 = x-10
Step 2: Eliminate the radical on the left hand side
Raise both sides to the second power
(√x-4)2 = (x-10)2
After squaring
x-4 = x2-20x+100
Step 3: Solve the quadratic equation
Rearranged equation
x2 - 21x + 104 = 0
This equation has two rational roots:
{x1, x2}={13, 8}
Step 4: Check that the first solution is correct
Original equation
√x-4 = x-10
Plug in 13 for x
√(13)-4 = (13)-10
Simplify
√9 = 3
Solution checks !!
Solution is:
x = 13
Step 5: Check that the second solution is correct
Original equation
√x-4 = x-10
Plug in 8 for x
√(8)-4 = (8)-10
Simplify
√4 = -2
Solution does not check
2 ≠ -2
One solution was found :
x = 13