Question

Part A. For which values of S and Mis S + M a rational number?

o
S=
13 and M=
1
16
O S =
Ja and Math
O S =
and M=
10
O SE 126 and M=
/
Pause

Answer 1

`B.\ (1)/(√(4) ) \ and \ (1)/(√(9) )`

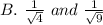
Explanation:

We need to know that rational numbers are numbers that can eb written as a ratio of two integers.

Given

S =
`(1)/(√(4) ) \ and \ M = (1)/(√(9) )`

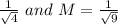
Add

Since √4 = 2 and √9 = 3

`S = (1)/(2) \ and \ M = (1)/(3)`

`S+M = (1)/(2) + (1)/(3)\\S+M = (3+2)/(6)\\S+M = (5)/(6)`

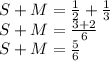
Since S+M is written as a ratio of two integers, hence the value of S and M for which S+M are rational is
`(1)/(√(4) ) \ and \ (1)/(√(9) )` respectively