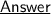
The given sequence is in Arithmetic progression, and we have to find its nth term ~
So, let's get it solved ~
First term of the sequence is :
Common difference is :
Now, if we have to write the 2nd Term with respect to first one, we can write :
- 2nd Term = a + (2 - 1)d = a + d = 2 + 2 = 4
similarly ~
- 3rd Term = a + (3 - 1)d = a + 2d = 2 + 4 = 6
- 4th Term = a + (4 - 1)d = a + 3d = 2 + 6 = 8
Therefore, I similar pattern ~
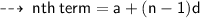
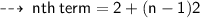
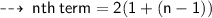
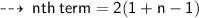
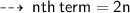
Feel free to ask your doubts, if you have any ~