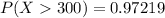Answer:
The probability is
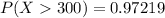
Explanation:
From the question we are told that
The capacity of an Airliner is k = 300 passengers
The sample size n = 320 passengers
The probability the a randomly selected passenger shows up on to the airport

Generally the mean is mathematically represented as
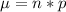
=>
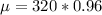
=>
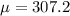
Generally the standard deviation is
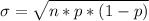
=>

=>
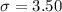
Applying Normal approximation of binomial distribution
Generally the probability that there will not be enough seats to accommodate all passengers is mathematically represented as
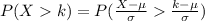
Here
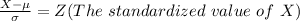
=>
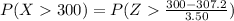
Now applying continuity correction we have
![P(X >300 ) = P(Z > ([300+0.5] - 307.2)/(3.50) )](https://img.qammunity.org/2021/formulas/mathematics/college/mvqj5jmhk8y00xn808g213u4ldjgwcc4rp.png)
=>
![P(X >300 ) = P(Z > ([300.5] - 307.2)/(3.50) )](https://img.qammunity.org/2021/formulas/mathematics/college/n05mh5h1lbk0noej7jwnjdbnogswz6p6nn.png)
=>

From the z-table
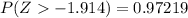
So