Answer:
The current in the loop is 10.5 A.
Step-by-step explanation:
Given that,
Radius = 9.4 cm
Magnetic field = 0.7 G
Angle = 70°
We know that,
The magnetic field due to the current in a loop is
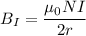
The magnetic field due to the current is equal to the magnetic field of earth.
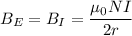
We need to calculate the current in the loop
Using formula of magnetic field
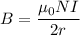
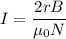
Put the value into the formula
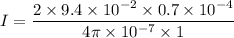
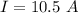
Hence, The current in the loop is 10.5 A.