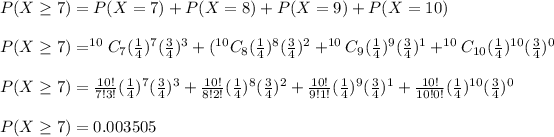Question

A student takes a multiple-choice test that has 10 questions. Each question has four choices. The student guesses randomly at each answer. Let X be the number of questions the student gets correct. (a) Find P(X = 3). (b) Find P(X > 2). (c) To pass the test, the student must answer 7 or more questions correctly. Would it be unusual for the student to pass? Explain.

Answer 1

1)0.2502

2)0.475

3)0.003505

Explanation:

Total No. of question n= 10

There are four choices in each question

So, Probability of success
`p = (1)/(4)`

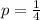
Probability of failure q =
`1- (1)/(4)=(3)/(4)`

We will use binomial over here

`P(X=x)=^nC_r p^r q^(n-r)`

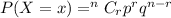
1)

`P(X = 3)=^(10)C_3 ((1)/(4))^3 ((3)/(4))^7\\P(X = 3)=(10!)/(3!7!) ((1)/(4))^3 ((3)/(4))^7\\P(X = 3)=0.2502`

2)
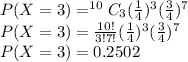
`P(X > 2)=1-P(X\leq 2)`

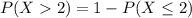
P(X>2)=1-(P(X=0)+P(X=1)+P(X=2))

`P(X>2)=1-(^(10)C_0 ((1)/(4))^0 ((3)/(4))^(10)+(^(10)C_1 ((1)/(4))^1 ((3)/(4))^9+^(10)C_2 ((1)/(4))^2 ((3)/(4))^8)`

`P(X>2)=1-(((1)/(4))^0 ((3)/(4))^(10)+((10!)/(1!9!) ((1)/(4))^1 ((3)/(4))^9+(10!)/(2!8!) ((1)/(4))^2 ((3)/(4))^8)`

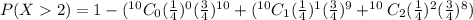
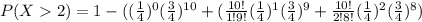
P(X>2)=0.475

3)

`P(X\geq 7)=P(X=7)+P(X=8)+P(X=9)+P(X=10)\\\\P(X\geq 7)=^(10)C_7 ((1)/(4))^7 ((3)/(4))^(3)+(^(10)C_8 ((1)/(4))^8 ((3)/(4))^2+^(10)C_9 ((1)/(4))^9 ((3)/(4))^1+^(10)C_(10) ((1)/(4))^(10) ((3)/(4))^0\\\\P(X\geq 7)=(10!)/(7!3!) ((1)/(4))^7 ((3)/(4))^(3)+(10!)/(8!2!) ((1)/(4))^8 ((3)/(4))^2+(10!)/(9!1!) ((1)/(4))^9 ((3)/(4))^1+(10!)/(10!0!)((1)/(4))^(10) ((3)/(4))^0\\\\P(X\geq 7)=0.003505`