Explanation:
The given expression is:
12x + 8y = 28
Problem;
Express in slope-intercept format;
Solution:
The equation of a straight line is given as;
y = mx + c
where y is the y-coordinate
m is the slope
x is the x-coordinate
c is the intercept
To solve this problem simply express the given equation as that of a straight line.
12x + 8y = 28 in the form y = mx + c
12x + 8y = 28
take 12x to the other side;
8y = 28 - 12x
divide by 8 to isolate the y;
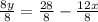
y =
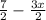
Re-arranging gives;
y =
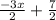
This is the slope-intercept form.