Question

5. The total number of people who went to Disneyland this weekend was

6,405. If the ratio of boys to girls is 7 to 9 for those that attended, what was
the TOTAL number of boys and girls that went to Disneyland this weekend?
Show your work or explain your thinking.

Answer 1

2,802 boys and 3,693 girls went to Disneyland this weekend

Explanation:

System of Equations

Let's call:

b = Number of boys who went to Disneyland this weekend.

g = Number of girls who went to Disneyland this weekend.

The first condition states there was a total of 6,405 people who went to Disneyland this weekend, thus:

`b+g=6,405\qquad\qquad [1]`

The second condition states that the ratio of boys to girls is 7 to 9. This can be written as:

`\displaystyle (b)/(g)=(7)/(9)\qquad\qquad [2]`

To eliminate denominators, multiply [2] by 9g:

`\displaystyle (9g)(b)/(g)=(9g)(7)/(9)`

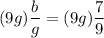
Simplify:

`9b=7g\qquad\qquad [3]`

From [1]:

`b=6,405-g`

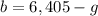
Substituting into [3]

`9(6,405-g)=7g`

Operating:

`57,645-9g=7g`

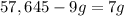
Simplify:

`16g=57,645`

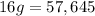
Solve:

`g=57,645 / 16 \approx 3,603`

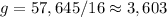
Take the approximate value:

g=3,603

Find b:

`b=6,405-3,603=2,802`

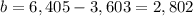
2,802 boys and 3,693 girls went to Disneyland this weekend

Note: Due to the nature of the problem, the results had to be rounded to the nearest integer. This condition makes the ratio not being exactly as required.