Question

A freshly prepared sample of radioactive isotope has an activity of 10 mCi. After 4 hours, its activity is 8 mCi. Find: (a) the decay constant  and half-life T1/2; (b) How many atoms of the isotope were contained in the freshly prepared sample? (c) What is the sample's activity 30 hours after it is prepared?

Answer 1

(a). The decay constant is
`1.55*10^(-5)\ s^(-1)`

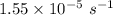
The half life is 11.3 hr.

(b). The value of N₀ is
`2.38*10^(11)\ nuclei`

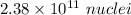
(c). The sample's activity is 1.87 mCi.

Step-by-step explanation:

Given that,

Activity
`R_(0)=10\ mCi`

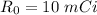
Time
`t_(1)=4\ hours`

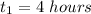
Activity R= 8 mCi

(a). We need to calculate the decay constant

Using formula of activity

`R=R_(0)e^(-\lambda t)`

`\lambda=(1)/(t)ln((R_(0))/(R))`

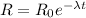
Put the value into the formula

`\lambda=(1)/(4*3600)ln((10)/(8))`

`\lambda=0.0000154\ s^(-1)`

`\lambda=1.55*10^(-5)\ s^(-1)`

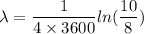
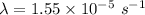
We need to calculate the half life

Using formula of half life

`T_{(1)/(2)}=(ln(2))/(\lambda)`

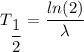
Put the value into the formula

`T_{(1)/(2)}=(ln(2))/(1.55*10^(-5))`

`T_{(1)/(2)}=44.719*10^(3)\ s`

`T_{(1)/(2)}=11.3\ hr`

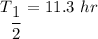
(b). We need to calculate the value of N₀

Using formula of
`N_(0)`

`N_(0)=(3.70*10^(6))/(\lambda)`

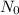
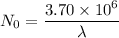
Put the value into the formula

`N_(0)=(3.70*10^(6))/(1.55*10^(-5))`

`N_(0)=2.38*10^(11)\ nuclei`

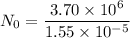
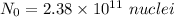
(c). We need to calculate the sample's activity

Using formula of activity

`R=R_(0)e^(-\lambda* t)`

Put the value intyo the formula

`R=10e^{-(1.55*10^(-5)*30*3600)}`

`R=1.87\ mCi`

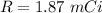
Hence, (a). The decay constant is
`1.55*10^(-5)\ s^(-1)`

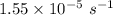
The half life is 11.3 hr.

(b). The value of N₀ is
`2.38*10^(11)\ nuclei`

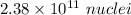
(c). The sample's activity is 1.87 mCi.