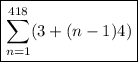Answer:
1) Σ [n=1, 418] (3+{n-1)4)
Explanation:
The expression for the sum is the sum over the number of terms of the expression for a general term.
__
general term
The general term of an arithmetic series is ...
an = a1 +d(n -1)
where a1 is the first term, and d is the common difference.
The given series has first term a1=3, and common difference d=7-3 = 4. Then the general term is ...
an = 3 +4(n -1)
number of terms
The last term will correspond to an n-value that can be found from ...
1671 = 3 +4(n -1)
1668 = 4(n -1) . . . . subtract 3
417 = n -1 . . . . . . . divide by 4
418 = n . . . . . . . add 1
sum expression
The expression for the sum is ...