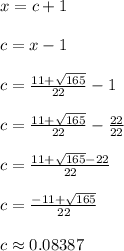Exact value:
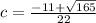
Approximate value: c = 0.08387
Round the approximate value however you need to
==============================================
Work Shown:
Let x = 1+c
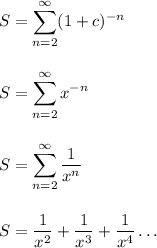
We have an infinite geometric series here. The first term is a = 1/(x^2). The common ratio is r = 1/x.
Each new term is found by multiplying the previous term by 1/x.
Assuming -1 < r < 1 is true, the infinite geometric sum is
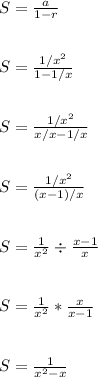
Plug in S = 11 and solve for x
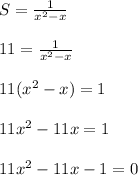
Use the quadratic formula to find the two solutions

Using these x values, we find that the corresponding r values are
r = 1/x = 1/(1.08387) = 0.92262
r = 1/x = 1/(-0.08387) = -11.92321
The first r value makes -1 < r < 1 true, but the second r value does not. So we will be ignoring the solution x = -0.08387
----------------------------------------------
Using the solution that corresponds to x = 1.08387, we find the value of c is